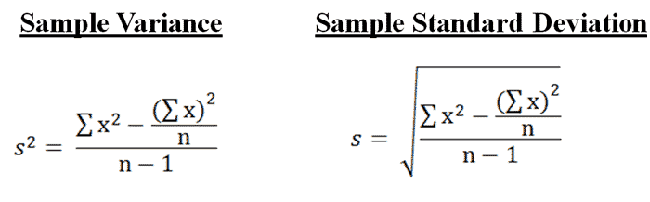
Computational Formula For Standard Deviation. S x2 x2 n n 1 s x 2 x 2 n n 1. N n i1 f i. Small Sample Example of Computational Formula for SS Variance and Standard Deviation. Compute the standard deviation of the following population once using the definitional formula and again using the computational formula.
S SSx where SSx Σx2 - Σx2 n 1 n. After this you have to subtract mean of each individual measurement and then take the square of a result. The formula for standard deviation depends on whether you are analyzing population data in which case it is called σ or estimating the population standard deviation from sample data which is. Standard Deviation Formula for Grouped Data. Similarly how do you find the standard deviation of a point. The sample standard deviation would tend to be lower than the real standard deviation of the population.
Compute the standard deviation of the following population once using the definitional formula and again using the computational formula.
Percent of Values Within 2 Standard Deviation. Standard Deviation There is a faster method of computing the standard deviation developed prior to the emergence of statistical software. First of all you have to calculate the mean by adding all individual data and then dividing all of them by the total number. Start by writing the computational formula for the standard deviation of a sample. The mid values of the classes are derived dividing the sum of upper and lower value of class and this value is used for calculations. Sigma sqrtfrac1Nsum_i1nf_ileftx_i-barxright2 Here N is given as.
