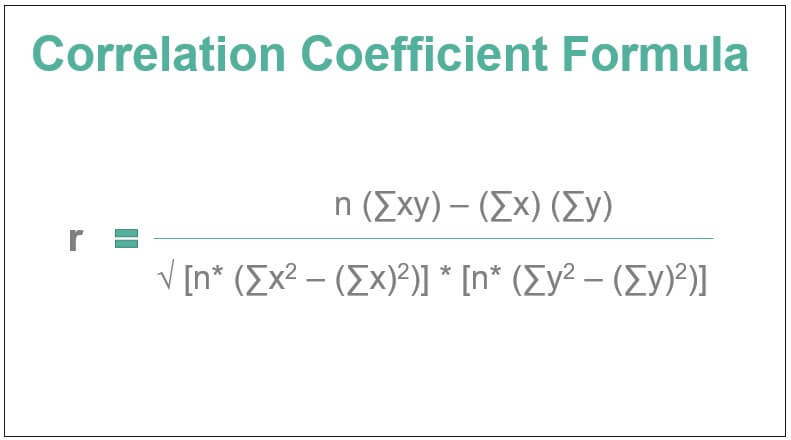
Multiple Correlation Coefficient Formula. X k then among all linear combinations of X 2. Where S x and S y are the sample standard deviations and S xy is the sample covariance. Coefficient Of Multiple Correlation. R xy S xy S x S y.
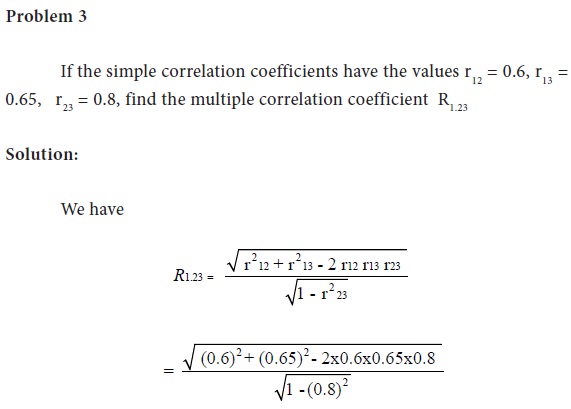
Sample size planning for multiple correlation. The given equation for correlation coefficient can be expressed in terms of means and expectations. In simple regression with one independent variable R 2 is simply the square of the correlation coefficient. The Output statement here is used to. Where r x z r y z and r x y are defined as the correlation coefficient between 2 variables. R 25 r 409 r 34 12 1Y 2Y 2 2409 34 - 2 409 34 25.
σX is the standard deviation of X and σY is the standard deviation of Y.
Coefficient Of Multiple Correlation. R xy S xy S x S y. R xy σ xy σ x σ y. If you have a correlation coefficient of -1 the rankings for one variable are the exact opposite of the ranking of the other variable. The given equation for correlation coefficient can be expressed in terms of means and expectations. Where S x and S y are the sample standard deviations and S xy is the sample covariance.